Finite-time Blow-up of Solutions of Some Long-wave Unstable Thin Film Equations

with A. L. Bertozzi, Indiana Univ. Math. J. 49(2000)4:1323-1366.

to appear in Indiana University Mathematics Journal

Abstract

We consider the family of long-wave unstable lubrication equations

ht = -(h hxxx)x - (hm hx)x

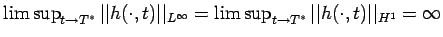with $m\geq 3$. Given a fixed $m\geq 3$, we prove the existence of a weak solution that becomes singular in finite time. Specifically, given compactly supported nonnegative initial data with negative energy, there is a time $T^* < \infty$, determined by m and the H1 norm of the initial data, and a compactly supported nonnegative weak solution such that $\limsup_{t\to T^*}\vert\vert h(\cdot, t)\vert\vert _{L^{\infty}} = \limsup_{t\to T^*}\vert\vert h(\cdot, t)\vert\vert _{H^1} = \infty$. We discuss the relevance of these singular solutions to an earlier conjecture [Comm Pure Appl Math 51:625-661, 1998] on when finite-time singularities are possible for long-wave unstable lubrication equations.



click here for the *.ps file of the article.
click here for the *.pdf file of the article.


We thank Andrew J. Bernoff for pointing out the formal second moment argument upon which the finite-time blow-up proof hinges. M. P. thanks Richard S. Laugesen for useful mathematical conversations.

A. B. was supported by an ONR Young Investigator/PECASE award and an Alfred P. Sloan Research Fellowship. M. P. was supported by NSF grant number DMS-9971392 and an Alfred P. Sloan Research Fellowship.