Day 1
u, v, w: topology and philosophy
- Dreams and plans.
- Knots, planar diagrams, Reidemeister moves, virtual knots are to knots as manifolds are to Euclidean spaces, flying rings and knotted tubes in 4D and w-knots.
- Planar algebras and circuit algebras.
- The abstract machine - filtered and graded spaces, expansions and homomorphic expansions, equations in graded spaces.
Day 2
u, v, w: combinatorics and low algebra
- Finite type invariants, weight systems, chord diagrams, arrow diagrams, 4T relations
- The "bracket-rise" theorem, STU and IHX relations
- Maps into various kinds of universal enveloping algebras.
- High algebra in the w case - from R4 to Kashiwara-Vergne - convolutions on Lie groups and algebras. (More in Talks: Bonn-0908).
Day 3
v: 18 Conjectures
- A w-Map for General Orientation (G3.html, G.pdf).
- I will state 18=3x3x2 "fundamental" conjectures on finite type invariants of various classes of virtual knots. This done, I will state a few further conjectures about these conjectures and ask a few questions about how these 18 conjectures may or may not interact.
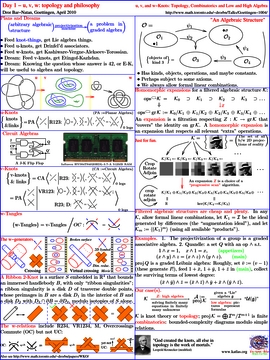
Handout - G1.html, G.pdf
 Talk Video.
Talk Video.
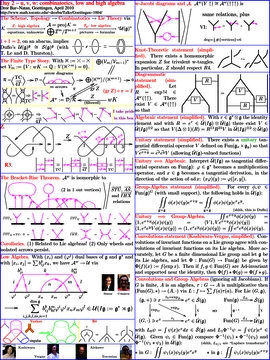
Handout - G2.html, G.pdf
 Talk Video.
Talk Video.
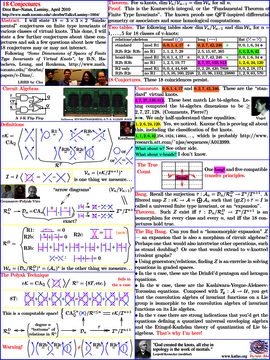
Handout - 18C.html, 18C.pdf
 Talk Video.
Talk Video.