Navigation Panel: 







 (These buttons explained below)
(These buttons explained below)
Course Outline
- Instructor:
- P. Spencer
- Office:
- South Building, Room 4063
phone: (905-828)-3809
email: spencer@math.toronto.edu
- Office Hours:
- Tuesday 2-3; Thursday 10:30-11:30, 3-4
- Textbook:
-
Anton & Rorres, Elementary Linear Algebra--Applications Version.
- Lectures:
-
Tuesday and Thursday, 12-1, South Building, Room
2074
- Marking Scheme:
-
|
Quizzes | 20% |
|
Midterm | 30% |
|
Final Exam | 50%
|
- Each week a problem set will be assigned. These are not to be
turned in.
- There will be a short quiz most weeks during tutorial. This will
consist of one of the questions from the previous week's problem set,
with a few details changed.
- Students who, for a valid reason, are unable to write one of the
quizzes should arrange beforehand with the tutor to write the quiz during
one of the other tutorial sections that same week.
- There will be one midterm, scheduled
for Thursday October 23rd.
- Any student who is too ill to write the midterm must present a
valid doctor's certificate within the following week. Depending on the
number of students and scheduling considerations, such students will either
be given a makeup midterm or else will have their midterm mark determined
from their other work in the course (a weighted average of quiz and final
exam marks, in the same proportion as above, with the final exam counting
2.5 times as much as the total of the quizzes).
- There will be no quiz or tutorials during the first week of
classes, and no quiz in the weeks of Sept. 15-19, Oct. 13-17, or Oct.
20-24.
We will cover in detail the material from chapters 1, 2, 4, 5, and 7:
Systems of Linear Equations, Matrices, Determinants, Euclidean and General
Vector Spaces, Linear Transformations in Euclidean Space, Eigenvalues, and
Eigenvectors.
Chapter 3 (Vectors in  and
and 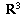 ;
review for most students) will be
summarized briefly; read this on your own. We will cover a selection of
topics from Chapter 6 (Orthogonality,
Gram-Schmidt Process, Least-Squares
Fit). Chapter 8 (General Linear Transformations) will
be covered earlier
and less formally than in the text, in conjunction with chapters 1 and 4.
;
review for most students) will be
summarized briefly; read this on your own. We will cover a selection of
topics from Chapter 6 (Orthogonality,
Gram-Schmidt Process, Least-Squares
Fit). Chapter 8 (General Linear Transformations) will
be covered earlier
and less formally than in the text, in conjunction with chapters 1 and 4.
We may cover a topic or two from Chapter 11 (Applications)
as time permits;
you are encouraged to browse the rest of that chapter on your own to get an
idea of how Linear Algebra is used in a wide variety of fields.
Navigation Panel: 








 Go up to Contents
Go up to Contents
 Go forward to Problem Set 1: Sept 15-19
Go forward to Problem Set 1: Sept 15-19
 Switch to text-only version (no graphics)
Switch to text-only version (no graphics)
 and
and 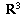 ;
review for most students) will be
summarized briefly; read this on your own. We will cover a selection of
topics from Chapter 6 (Orthogonality,
Gram-Schmidt Process, Least-Squares
Fit). Chapter 8 (General Linear Transformations) will
be covered earlier
and less formally than in the text, in conjunction with chapters 1 and 4.
;
review for most students) will be
summarized briefly; read this on your own. We will cover a selection of
topics from Chapter 6 (Orthogonality,
Gram-Schmidt Process, Least-Squares
Fit). Chapter 8 (General Linear Transformations) will
be covered earlier
and less formally than in the text, in conjunction with chapters 1 and 4.