Talk I:
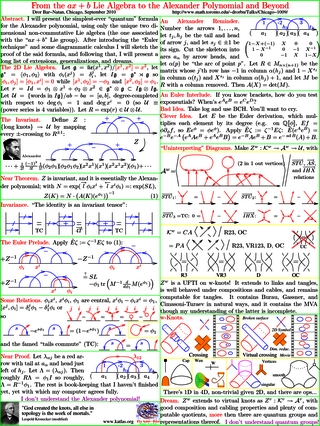
From the ax+b Lie Algebra to the Alexander Polynomial and Beyond
Abstract.
I will present the simplest-ever "quantum" formula for the Alexander
polynomial, using only the unique two dimensional non-commutative Lie algebra
(the one associated with the "ax+b" Lie group). After introducing
the "Euler technique" and some diagrammatic calculus I will sketch the
proof of the said formula, and following that, I will present a long
list of extensions, generalizations, and dreams.
Handout: axpb.html, axpb.pdf.
Source Files: axpb.zip.
 Talk
Video. Talk
Video.
See also Finite Type Invariants of
w-Knotted Objects: From Alexander to Kashiwara and Vergne, and Talks: Toronto-1005.
|
 |
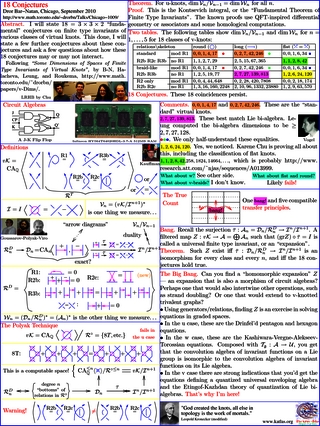
Talk II: 18 Conjectures
Abstract.
I will state 18=3x3x2 "fundamental" conjectures on finite type invariants of
various classes of virtual knots. This done, I will state a few further
conjectures about these conjectures and ask a few questions about how these 18
conjectures may or may not interact.
Handout: 18C.html, 18C.pdf.
Source Files: 18C.zip.
 Talk
Video. Talk
Video.
See also Some Dimensions of
Spaces of Finite Type Invariants of Virtual Knots, with Halacheva,
Leung, and Roukema, and Talks:
Goettingen-1004.
|
 |
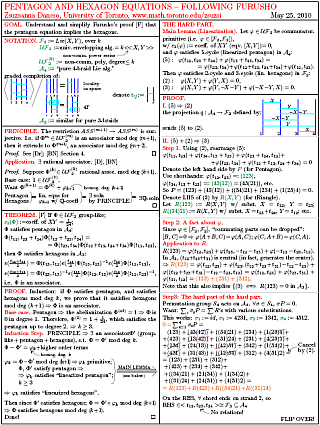
Dancso's Talk: Pentagon and Hexagon Equations - Following
Furusho
Abstract.
In his beautiful paper 'Pentagon and hexagon equations' (Annals
Math., Vol 171 (2010), No.1, 545-556), H. Furusho proves that of the
defining equations for associators, the pentagon equation implies the
hexagons. After a brief introduction to associators, we will sketch a
simplified proof. In particular, we replace the use of algebraic geometry
by the fact that associators up to a given degree can be extended to
the next degree, and eliminate the use of spherical braids.
Handout: furusho.pdf.
 Talk
Video. Talk
Video.
See also Zsuzsanna Dancso's home page.
|
 |



 Talk
Video.
Talk
Video.