



Next: References beginning with U
Up: References
Previous: References beginning with S
Contents
References beginning with T
 K. Taniyama and A. Yasuhara,
Band description of knots and Vassiliev invariants,
to appear in Mathematical Proceedings of the Cambridge Philosophical
Society,
arXivmath.GT/0003021.
K. Taniyama and A. Yasuhara,
Band description of knots and Vassiliev invariants,
to appear in Mathematical Proceedings of the Cambridge Philosophical
Society,
arXivmath.GT/0003021.
 K. Taniyama and A. Yasuhara,
Local moves on spatial graphs and finite type invariants,
Pac. Jour. of Math., to appear,
arXiv:math.GT/0106173.
K. Taniyama and A. Yasuhara,
Local moves on spatial graphs and finite type invariants,
Pac. Jour. of Math., to appear,
arXiv:math.GT/0106173.
 P. Teichner,
Knots, von Neumann signatures, and grope cobordism,
Proceedings of the ICM, Beijing 2002, 2 437-446,
arXiv:math.GT/0304299.
P. Teichner,
Knots, von Neumann signatures, and grope cobordism,
Proceedings of the ICM, Beijing 2002, 2 437-446,
arXiv:math.GT/0304299.
 G. Thompson,
Holomorphic vector bundles, knots and the Rozansky-Witten
invariants,
ICTP Trieste preprint IC/2000/9, February 2000. See also
hep-th/0002168.
G. Thompson,
Holomorphic vector bundles, knots and the Rozansky-Witten
invariants,
ICTP Trieste preprint IC/2000/9, February 2000. See also
hep-th/0002168.
 D. Thurston,
Integral expressions for the Vassiliev knot invariants,
Harvard University senior thesis, April 1995, arXiv:math.QA/9901110.
D. Thurston,
Integral expressions for the Vassiliev knot invariants,
Harvard University senior thesis, April 1995, arXiv:math.QA/9901110.
 D. Thurston,
Wheeling: A diagrammatic analogue of the Duflo isomorphism,
University of California at Berkeley Ph.D. thesis, May 2000,
arXiv:math.QA/0006083.
D. Thurston,
Wheeling: A diagrammatic analogue of the Duflo isomorphism,
University of California at Berkeley Ph.D. thesis, May 2000,
arXiv:math.QA/0006083.
 D. Thurston,
The algebra of knotted trivalent graphs and Turaev's shadow
world,
Invariants of knots and 3-manifolds (Kyoto 2001), Geometry and
Topology Monographs 4 337-362, arXiv:math.GT/0311458.
D. Thurston,
The algebra of knotted trivalent graphs and Turaev's shadow
world,
Invariants of knots and 3-manifolds (Kyoto 2001), Geometry and
Topology Monographs 4 337-362, arXiv:math.GT/0311458.
 V. Tourtchine,
Sur l'homologie des espaces de n
V. Tourtchine,
Sur l'homologie des espaces de n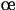 uds non-compacts,
Independent Moscow University and Université de Paris 7 preprint,
October 2000,
arXiv:math.QA/0010017.
uds non-compacts,
Independent Moscow University and Université de Paris 7 preprint,
October 2000,
arXiv:math.QA/0010017.
 V. Tourtchine,
On the homology of the spaces of long knots,
Independent Moscow University and Université de Paris 7 preprint,
May 2001,
arXiv:math.QA/0105140.
V. Tourtchine,
On the homology of the spaces of long knots,
Independent Moscow University and Université de Paris 7 preprint,
May 2001,
arXiv:math.QA/0105140.
 V. Tourtchine,
On the other side of the bialgebra of chord diagrams,
Independent Moscow University and Université Catholique de Louvain
preprint, November 2004, arXiv:math.QA/0411436.
V. Tourtchine,
On the other side of the bialgebra of chord diagrams,
Independent Moscow University and Université Catholique de Louvain
preprint, November 2004, arXiv:math.QA/0411436.
 R. Trapp,
Twist sequences and Vassiliev invariants,
Jour. of Knot Theory and its Ramifications 3(3) (1994) 391-405.
R. Trapp,
Twist sequences and Vassiliev invariants,
Jour. of Knot Theory and its Ramifications 3(3) (1994) 391-405.
 V. Turchin,
Homology isomorphism of the complex of 2-connected graphs and the
graph-complex of trees,
in Topics in quantum groups and finite-type invariants,
(B. Feigin and V. Vassiliev, eds.) AMS Translations Ser. 2
vol. 185 (1998) 147-153, Providence.
V. Turchin,
Homology isomorphism of the complex of 2-connected graphs and the
graph-complex of trees,
in Topics in quantum groups and finite-type invariants,
(B. Feigin and V. Vassiliev, eds.) AMS Translations Ser. 2
vol. 185 (1998) 147-153, Providence.
 V. Turchin,
Calculus of the first non-trivial 1-cocycle of the space of long
knots,
Independent University of Moscow preprint, February 2005,
arXiv:math.AT/0502518.
V. Turchin,
Calculus of the first non-trivial 1-cocycle of the space of long
knots,
Independent University of Moscow preprint, February 2005,
arXiv:math.AT/0502518.
 S. D. Tyurina,
On formulas of the Lannes and Viro-Polyak type for the finite
degree invariants,
Mat. Zametki 4(66) (1999), arXiv:math.AT/9905160.
S. D. Tyurina,
On formulas of the Lannes and Viro-Polyak type for the finite
degree invariants,
Mat. Zametki 4(66) (1999), arXiv:math.AT/9905160.
 S. D. Tyurina,
Explicit formulas for the Vassiliev knot invariants,
Kolomensky Pedagogical Institute preprint, May 1999. See also
math.AT/9905161.
S. D. Tyurina,
Explicit formulas for the Vassiliev knot invariants,
Kolomensky Pedagogical Institute preprint, May 1999. See also
math.AT/9905161.
 S. D. Tyurina,
Diagram formulas of the Viro-Polyak type for finit degree
invariants,
Russian Mathematical Surveys 54(3) (1999) 658-659,
arXiv:math.AT/9905162.
S. D. Tyurina,
Diagram formulas of the Viro-Polyak type for finit degree
invariants,
Russian Mathematical Surveys 54(3) (1999) 658-659,
arXiv:math.AT/9905162.
 S. D. Tyurina,
The Kontsevich integral for
S. D. Tyurina,
The Kontsevich integral for 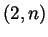 -type torus knots,
Proc. Rokhlin Memorial conference, Euler Institute, Saint-Petersburg,
August 1999.
-type torus knots,
Proc. Rokhlin Memorial conference, Euler Institute, Saint-Petersburg,
August 1999.
 S. D. Tyurina and A. Varchenko,
A remark on
S. D. Tyurina and A. Varchenko,
A remark on 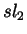 approximation of the Kontsevich integral of the
unknot,
Zapiski Nauchnyh Seminarov POMI 299 30-37, arXiv:math.AT/0111201
approximation of the Kontsevich integral of the
unknot,
Zapiski Nauchnyh Seminarov POMI 299 30-37, arXiv:math.AT/0111201
 S. D. Tyurina and A. Varchenko,
Finite order invariants for
S. D. Tyurina and A. Varchenko,
Finite order invariants for 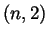 -torus knots and the curve
-torus knots and the curve
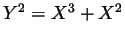 ,
University of North Carolina at Chapel Hill preprint, February 2004,
arXiv:math.GT/0402185.
,
University of North Carolina at Chapel Hill preprint, February 2004,
arXiv:math.GT/0402185.




Next: References beginning with U
Up: References
Previous: References beginning with S
Contents
Dror Bar-Natan
2005-06-30