International Mathematical Talent Search
International Mathematical Talent Search
 for all real numbers
for all real numbers  for which 19x + 95y = 1995.
for which 19x + 95y = 1995.
Problem 2/18. For a positive integer 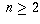 , let P(n)
denote the product of the positive integer divisors (including 1 and n)
of n. Find the smallest n for which
, let P(n)
denote the product of the positive integer divisors (including 1 and n)
of n. Find the smallest n for which  .
.
Problem 3/18. The graph shown below has 10 vertices, 15 edges, and each vertex is of order 3 (i.e., at each vertex 3 edges meet). Some of the edges are labelled 1, 2, 3, 4, 5 as shown. Prove that it is possible to label the remaining edges 6, 7, 8, . . . , 15 so that at each vertex the sum of the labels on the edges meeting at that vertex is the same.
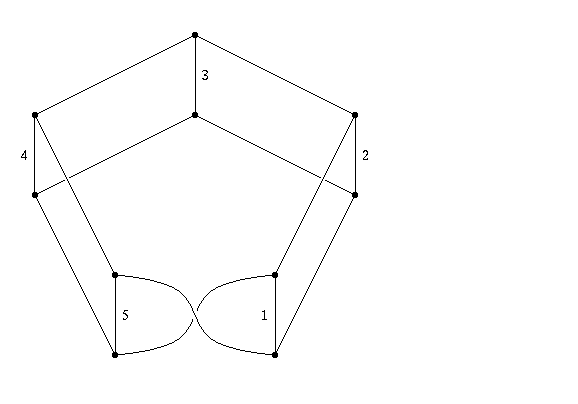
Problem 4/18. Let a, b, c, d be distinct real numbers
such that a+b+c+d = 3 and  .
Find the value of the expression
.
Find the value of the expression
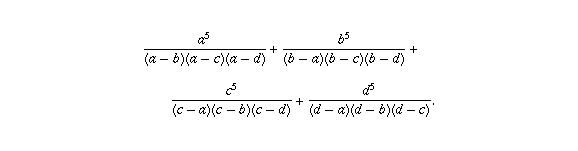
Problem 5/18. Let a and b be two lines in the plane, and let C be a point, as shown below. Using only a compass and an unmarked straightedge, construct an isosceles right triangle ABC, so that A is on line a, B is on line b, and AB is the hypotenuse of triangle ABC.
Solve as many of the problems as you can (you need not solve them all), and mail your solutions to:
Professor E. J. BarbeauMake sure that the front page of your solutions contains your full name and mailing address.
Department of Mathematics
University of Toronto
Toronto, ON M5S 3G3
![]() Go backward to Round 17
Go backward to Round 17![]() Go up to Talent Search Index
Go up to Talent Search Index![]() Go forward to Round 19
Go forward to Round 19![]() Switch to text-only version (no graphics)
Switch to text-only version (no graphics)![]() Access printed version in PostScript format (requires PostScript printer)
Access printed version in PostScript format (requires PostScript printer)![]() Go to University of Toronto Mathematics Network
Home Page
Go to University of Toronto Mathematics Network
Home Page